Sets

• Dixon999

  26 Dec 08, 11:15

  wonder if there's ani difference between subset n proper subset? when 2 use subset n when 2 use proper subset to represent or can they be used interchangeably...can ani1 clear my doubts???

• Arkansaw

  26 Dec 08, 11:16

  first do you even know what is meant by proper subset

• eagle

  26 Dec 08, 11:31

  From Wiki, I bolded the important part

   

  If A and B are sets and every element of A is also an element of B, then:
          * A is a subset of (or is included in) B, denoted by .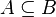
  
  If A is a subset of B, but A is not equal to B (i.e. there exists at least one element of B not contained in A), then
          * A is also a proper (or strict) subset of B; this is written as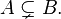
  
  
  

• wee_ws

  26 Dec 08, 12:47

  Hi,

    Interestingly, I have to use the concept of sets often in my research :)

    Study sets well if you need to study mathematics at the university level!

    Happy new year!

  Cheers,
  Wen Shih

• wee_ws

  26 Dec 08, 17:38

  Hi,

    In my research, I deal with filters.

    A filter is a non-empty set whose members are supersets of a particular given set. As an example, consider the set X = {1, 2, 3}. The filter generated by the set {1} has the following members: {1}, {1, 2}, {1, 3} and X.

    Note that any filter is a subet of the powerset of X, whose members are the empty set, {1}, {2}, {3}, {1, 2}, {1, 3}, {2, 3} and X.

    Thanks!

  Cheers,
  Wen Shih