tys e maths ques

• crucifiedx

  22 Oct 08, 11:57

  The temperature at 0900 is -4 degree celsius.

  The temperature at 1500 is 14 degree celsius.

   

  Assuming that the temperature rises at a steady rate, find

  (i) the temperature at 1300,

  (ii) the time when the temperature is 12.5 degree celsius.

   

  for (i), why can't i do like that:

  6h - 18 degree celcius

  1h - 3 degree celsius

  4h - 12 degree celsius

  at 1300, -4+12=8 degree celcius?

• crucifiedx

  22 Oct 08, 12:43

  and this:

   

  y is directly proportional to x^2.

  It is known that y=10 for a particular value of x.

  Find the value of y when this value of x is halved.

• davidche

  22 Oct 08, 12:45

  9 am to 1pm ish how many hours?

   

• skythewood

  22 Oct 08, 12:51

   y = x^2

  when y = 10, x = square root (10)

  half this, x = [square root (10)] / 2

      x^2 = 10 /4

            = 2.5

   

  so y is 2.5

• davidche

  22 Oct 08, 12:54

  Originally posted by crucifiedx:

  and this:

   

  y is directly proportional to x^2.

  It is known that y=10 for a particular value of x.

  Find the value of y when this value of x is halved.

  y = kx^2

   

  k(x/2)^2 = kx^2 /4 = 1/4 * kx^2

  Thus when x is halfed, y is ''quatered''.

  haven finish 1/4 *kx^2 = 1/4 y = 1/4 (10) = 2.5

• crucifiedx

  22 Oct 08, 13:09

  Originally posted by davidche:

  9 am to 1pm ish how many hours?

   

  
  4 hrs.

• eagle

  22 Oct 08, 13:11

  Originally posted by crucifiedx:

  The temperature at 0900 is -4 degree celsius.

  The temperature at 1500 is 14 degree celsius.

   

  Assuming that the temperature rises at a steady rate, find

  (i) the temperature at 1300,

  (ii) the time when the temperature is 12.5 degree celsius.

   

  for (i), why can't i do like that:

  6h - 18 degree celcius

  1h - 3 degree celsius

  4h - 12 degree celsius

  at 1300, -4+12=8 degree celcius?

  I don't see anything wrong with that

• crucifiedx

  22 Oct 08, 13:16

  i think ans wrong then.. part (ii) how to do?

• eagle

  22 Oct 08, 13:18

  Originally posted by crucifiedx:

  i think ans wrong then.. part (ii) how to do?

  12.5 degrees celcius, means 16.5 degrees change

  Continue with your workings

  1h - 3 degree celsius
  1/6 h - 0.5 degree celsius
  33/6 hrs - 16.5 degrees

  33/6 hours = 5.5 hours

  so 0900 + 5.5 hrs = 1430

• maurizio13

  22 Oct 08, 13:21

   

  does e maths have naperian logarithm?

   

   

• crucifiedx

  22 Oct 08, 13:22

  Originally posted by eagle:

  12.5 degrees celcius, means 16.5 degrees change

  Continue with your workings

  1h - 3 degree celsius
  1/6 h - 0.5 degree celsius
  33/6 hrs - 16.5 degrees

  33/6 hours = 5.5 hours

  so 0900 + 5.5 hrs = 1430

  
  i forgot to add the -4 in my first step. thanks alot. =D

• eagle

  22 Oct 08, 13:31

  good good...

  think you can near B3 to A2 this time round... work hard today and tomorrow

• crucifiedx

  22 Oct 08, 13:38

  i hope so.. haha. tml can have tuition at 7.30?

• eagle

  22 Oct 08, 13:47

  should be ok

• crucifiedx

  22 Oct 08, 13:53

  ok.. i got another ques:

   

  A regular polygon has n sides. The size of each interior angle is 9 times the size of each exterior angle.

   

  (i) calculate the size of each exterior angle.

  (ii) hence, find the value of n.

• crucifiedx

  22 Oct 08, 13:55

  and this: write down the positive square root of 25a^8.

   

  and:

   

  the two sides of a right-angled triangle are of length 1cm and 2cm respectively, find the two possible values of the third side. Leave your answer in the form square root a, where a is a positive integer. 

   

  I don't have the answers..

• eagle

  22 Oct 08, 13:57

  Originally posted by crucifiedx:

  and this: write down the positive square root of 25a^8.

   

  i don't have the answers..

  25a^8

  = (5a^4)^2

  so square root gives you 5a^4

   

  Indices => for square root, take the power divide by 2

• skythewood

  22 Oct 08, 13:59

  Originally posted by crucifiedx:

  ok.. i got another ques:

   

  A regular polygon has n sides. The size of each interior angle is 9 times the size of each exterior angle.

   

  (i) calculate the size of each exterior angle.

  (ii) hence, find the value of n.

  interior plus exterior angle = 360?

• eagle

  22 Oct 08, 14:00

  Originally posted by crucifiedx: ok.. i got another ques:

   

  A regular polygon has n sides. The size of each interior angle is 9 times the size of each exterior angle.

   

  (i) calculate the size of each exterior angle.

  (ii) hence, find the value of n.

  (i) interior angle + exterior angle = 180°
  => think you can do le

  (ii) size of each exterior angle is 360° / n
  Use answer from (i) to help solve

• skythewood

  22 Oct 08, 14:05

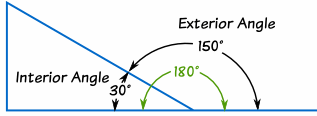
  ooo... the whole angle outside is the reflex angle, not exterior angle.

• eagle

  22 Oct 08, 14:09

  Originally posted by skythewood:

  ooo... the whole angle outside is the reflex angle, not exterior angle.

  yup

  for clarity

  

• crucifiedx

  22 Oct 08, 14:12

  thanks.. =)

   

  this ques how to do ar?

   

  the two sides of a right-angled triangle are of length 1cm and 2cm respectively, find the two possible values of the third side. Leave your answer in the form square root a, where a is a positive integer. 

   

  is the ans 5?

• skythewood

  22 Oct 08, 14:16

  two possibilties

  the unknown is the hypotenuse

  or 2 cm is the hypotenuse

• crucifiedx

  22 Oct 08, 14:19

  Originally posted by skythewood:

  two possibilties

  the unknown is the hypotenuse

  or 2 cm is the hypotenuse

  
  then how to solve..?

• eagle

  22 Oct 08, 14:20

  Originally posted by crucifiedx:

  thanks.. =)

   

  this ques how to do ar?

   

  the two sides of a right-angled triangle are of length 1cm and 2cm respectively, find the two possible values of the third side. Leave your answer in the form square root a, where a is a positive integer. 

   

  is the ans 5?

  There are 2 values

  1) Consider 2 as the hypotenuse
  side will be sqrt(2^2 - 1^2) = sqrt(3)

  2) Consider final side as hypotenuse
  side will be sqrt (2^2 + 1^2) = sqrt(5)

  so a can be 3 or 5