Pri 6 maths questions
-
Hey guys, as seen in the prev thread which I started in this forums, I am coaching my cousin for her upcoming PSLE. However, there are some questions which I myself couldn't answer! Hope you guys can help!
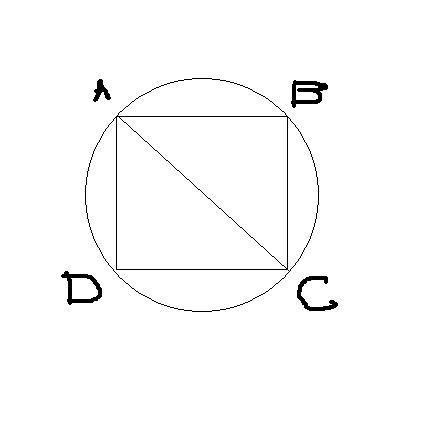
Q1) Area of SQ = 70cm2, what is the area of circle?
Q2) Sally bought some identical red files. Cost of each file = $1.40
When she bought 1 more blue file at $4.60, average cost becomes $1.80
How many files did Sally buy altogether? -
Originally posted by technoboy:
Hey guys, as seen in the prev thread which I started in this forums, I am coaching my cousin for her upcoming PSLE. However, there are some questions which I myself couldn't answer! Hope you guys can help!
Q1) Area of SQ = 70cm2, what is the area of circle?

Q2) Sally bought some identical red files. Cost of each file = $1.40
When she bought 1 more blue file at $4.60, average cost becomes $1.80
How many files did Sally buy altogether?
area of two square will be 140cm2therefore diameter will be square root (140)
area of circle = pi r^2 = 3.14 * root (140)/2 * root (140)/2 = 3.14 * 35 = 109.96cm2
Q2) Let the number of red files she bought be x
1.40x + 4.60 = 1.8 (x+1)
1.4x + 4.60 = 1.8x + 1.8
0.4x = 2.8
x=7She bought 7+1 = 8 files altogether.
-
you see the square is made of 2 triangle?
now, use four of these triangle to make another square.
find diameter, find radius, find answer
-
Sorry, I still don't quite get the first question. Where should I place the other sq which I draw myself?
Getting real rusty...
-
Originally posted by technoboy:
Sorry, I still don't quite get the first question. Where should I place the other sq which I draw myself?
Getting real rusty...
break the square into 2 triangles....
break 2nd square into 2 triangles
combine this 4 triangles... to form a square....
the square should have the diagonal of the original as it's side instead.
-
formula of area of any square = (diagonal^2)/2 in this question, diagonal of square = diameter of circle.
-
Originally posted by FirePig:
formula of area of any square = (diagonal^2)/2
in this question, diagonal of square = diameter of circle.
didn't know that. kids learn this in primary school now?
gues i'm outdated le
-
Area of SQ = 70cm2 as given
i.e. (AB x AB) or (BC x BC) = 70cm2
Pythegoras Theorem,
AC x AC = (AB x AB) + (BC x BC)
-
Area of SQ = 70cm2 as given
i.e. (AB x AB) or (BC x BC) = 70cm2
Pythegoras Theorem,
AC x AC = (AB x AB) + (BC x BC)
= 70 + 70
= 140
therefore AC = square root of 140 which is also the radius of circle
Area of circle = (22/7) x radius x radius
= 22/7 x AC x AC
= 22/7 x 140/4
= 110cm2
Got it? I will reply to the 2nd Q soon.
-
Originally posted by skythewood:
didn't know that. kids learn this in primary school now?
gues i'm outdated le
actually hor, I learned about pygathoras theorem in pri sch
-
Originally posted by eagle:
actually hor, I learned about pygathoras theorem in pri sch
Core syllabus or self study? -
Guys I have a question, since all of you here gave the answer which requires square root, how are they suppose to calculate when they do not have the calculator?
Can they just leave it in the sq rt form?
-

-
Wtf? Why pri sch question now so freaking difficult one? I cannot solve it using pri sch math leh!
I'm not sure if we should solve the first question using pygathoras theorem. I learnt it only in sec 2.
Pri sch kids do learn algebra...but have they learnt changing the subject of algebric equation? If not, then no point using algebra because it will involve changing subject.
Baiyun's method will work for a pri school kid...it's shameful that I did not think of his method, lol.
but what about the second question?
-
the first question don't need pythygoras nia...
use this, which i also didn't learn in primary school
formula of area of any square = (diagonal^2)/2
in this question, diagonal of square = diameter of circle.
-
2nd question use algebra loh
-
Hey guys, 2 more questions for you. Hope someone can help me with it!
Q1) There are 2 identical flights of stairs. The first flight, A walked some and ran 4 steps. he took 98 secs. The second flight, A walked some and ran 9 steps. He took 78 secs. How long does he need to walk both flights?
My answer: (please tell me if it's correct as I'm not too sure)
Running 5 steps instead of walking = 20 secs faster
Running 1 step instead of walking = 5 secs fasterThus, walk the whole flight = 98 + (4*4) = 114sec (1 flight of stairs)
Total timing to walk both flights = 114 * 2 = 228 secs
Q2) A piece of rectangular paper is folded as below. Find X and Y.
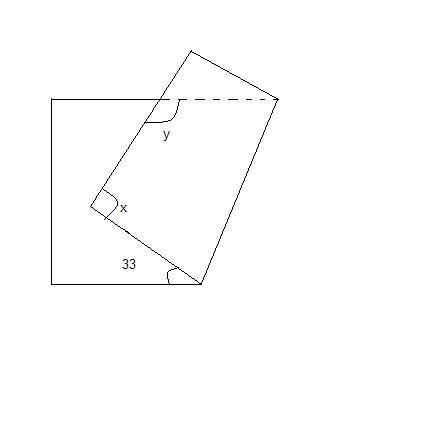
-
Originally posted by technoboy:
Hey guys, 2 more questions for you. Hope someone can help me with it!
Q1) There are 2 identical flights of stairs. The first flight, A walked some and ran 4 steps. he took 98 secs. The second flight, A walked some and ran 9 steps. He took 78 secs. How long does he need to walk both flights?
My answer: (please tell me if it's correct as I'm not too sure)
Running 5 steps instead of walking = 20 secs faster
Running 1 step instead of walking = 5 secs fasterThus, walk the whole flight = 98 + (4*4) = 114sec (1 flight of stairs)
Total timing to walk both flights = 114 * 2 = 228 secs
Q2) A piece of rectangular paper is folded as below. Find X and Y.

If I am not wrong, x is 90 degrees. Its the edge of the rectangle and therefore should be 90 degrees. As for y, I am still working it out.
-
 y= 123 DEGREES
y= 123 DEGREES -
corresponding angle, find the top right corner.
angle sum of triangle, find A.
angles on a straight line, line y.
-
actually a quicker methood. ext. angle of a triangle, y = 90 + 33 <sup>o</sup>
-
Originally posted by tr@nsp0rt_F3V3R:
actually a quicker methood. ext. angle of a triangle, y = 90 + 33 <sup>o</sup>
yes, but i don't like to teach my student ext. angle of triangle.
if they need me to teach them, they probably is having trouble le, so i see no point teaching them this step, which is just a combination of two steps. if they can handle the other 4 types of angle relation, is enough le.
-
Ok, last 3 questions guys before she goes for battle tomorrow!
These problems really give me an headache!
1) A cashier had 151 pieces of $50 notes and $10 notes. She took out 3/4 of the $50 notes and put in nine $10 notes. As a result, the ratio of the $50 to $10 becomes 3:8. What is the total value of the $10 notes initially?
2) The length of a bridge is 1500m. A train takes 150secs to travel on the bridge. The whole train was completely on the bridge for 100secs. Find (a) the average speed of the train and (b) the length of the train
3) P overlaps Q & R. Q is on R. Ratio of area of P, Q, R is 1:2:3. 40% of Q is shaded.Find the % of the figure unshaded.
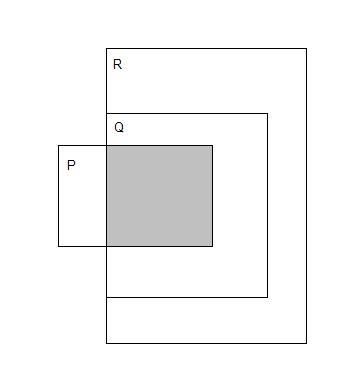
-
Originally posted by technoboy:
1) A cashier had 151 pieces of $50 notes and $10 notes. She took out 3/4 of the $50 notes and put in nine $10 notes. As a result, the ratio of the $50 to $10 becomes 3:8. What is the total value of the $10 notes initially?
presently,
$50 ===> X X X
$10 ===> X X X X X X X Xat the beginning, before taking away 3/4 of $50
$50 ===> X X X X X X X X X X X X
$10 ===> X X X X X X X X
number of notes is 151 + 9 = 160 (include the 9 $10)simplify diagram to
$50 ===> X X X
$10 ===> X Xone X is equal to 160/5 = 32 notes
hence, you can solve the thing
-
Originally posted by technoboy:
2) The length of a bridge is 1500m. A train takes 150secs to travel on the bridge. The whole train was completely on the bridge for 100secs. Find (a) the average speed of the train and (b) the length of the train
a) speed = distance divide by time
= 1500 / 150
= 10m/sb) the 100 seconds is the time between the end of the train get on the bridge, and the front of the train reach the end of the train.
distance travelled = speed x time
= 10 x 100
- 1000mhence, the train is = 1500 - 1000
= 500mbridge starts |< ------------------1500------------------------------------>| bridge ends
| train starts|<------------distance travelled---------->|
| train ends |